How To Get Frequency From Wavelength

Learning Objectives

Define wavelength.
Define frequency.
Describe the characteristics of a wave.
Perform calculations involving wavelength and frequency.

Do you enjoy going to the beach?

During the summer, almost everyone enjoys going to the beach. They can swim, have picnics, and work on their tans. But if you get too much sun, you can burn. A particular set of solar wavelengths are especially harmful to the skin. This portion of the soar spectrum is known as UV B, with wavelengths of 280–320 nm. Sunscreens are effective in protecting the skin against both the immediate skin damage and the long-term possibility of skin cancer.

UV light causes suntans at a beach

Waves

Waves are characterized by their repetitive motion. Imagine a toy boat riding the waves in a wave pool. As the water wave passes under the boat, it moves up and down in a regular and repeated fashion. While the wave travels horizontally, the boat only travels vertically up and down. The Figure below shows two examples of waves.

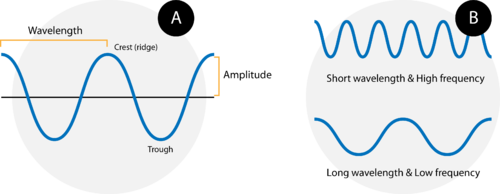
(A) A wave consists of alternation crests and troughs. The wavelength (CE-BB) is defined as the distance between any two consecutive identical points on the waveform. The amplitude is the height of the wave. (B) A wave with a short wavelength (top) has a high frequency because more waves pass a given point in a certain amount of time. A wave with a longer wavelength (bottom) has a lower frequency.

Figure 1. (A) A wave consists of alternation crests and troughs. The wavelength (λ) is defined as the distance between any two consecutive identical points on the waveform. The amplitude is the height of the wave. (B) A wave with a short wavelength (top) has a high frequency because more waves pass a given point in a certain amount of time. A wave with a longer wavelength (bottom) has a lower frequency.

A wave cycle consists of one complete wave—starting at the zero point, going up to a wave crest, going back down to a wave trough, and back to the zero point again. The wavelength of a wave is the distance between any two corresponding points on adjacent waves. It is easiest to visualize the wavelength of a wave as the distance from one wave crest to the next. In an equation, wavelength is represented by the Greek letter lambda (λ). Depending on the type of wave, wavelength can be measured in meters, centimeters, or nanometers (1 m = 10⁹ nm). The frequency, represented by the Greek letter nu (ν), is the number of waves that pass a certain point in a specified amount of time. Typically, frequency is measured in units of cycles per second or waves per second. One wave per second is also called a Hertz (Hz) and in SI units is a reciprocal second (s⁻¹).

Figure B above shows an important relationship between the wavelength and frequency of a wave. The top wave clearly has a shorter wavelength than the second wave. However, if you picture yourself at a stationary point watching these waves pass by, more waves of the first kind would pass by in a given amount of time. Thus the frequency of the first waves is greater than that of the second waves. Wavelength and frequency are therefore inversely related. As the wavelength of a wave increases, its frequency decreases. The equation that relates the two is:

c = λν

The variablec is the speed of light. For the relationship to hold mathematically, if the speed of light is used in m/s, the wavelength must be in meters and the frequency in Hertz.

Sample Problem: Wavelength and Frequency

The color orange within the visible light spectrum has a wavelength of about 620 nm. What is the frequency of orange light?

Step 1: List the known quantities and plan the problem.

Known

wavelength (λ) = 620 nm
speed of light (c) = 3.00 × 10⁸ m/s
conversion factor 1 m = 10⁹ nm

Unknown

Frequency

Convert the wavelength to m, then apply the equationc = λν and solve for frequency. Dividing both sides of the equation byλ yields:

[latex]\displaystyle\nu=\frac{c}{\lambda}[/latex]

Step 2: Calculate

[latex]\displaystyle620\text{ nm}\times\left(\frac{1\text{ m}}{10^9\text{ nm}}\right)=6.20\times10^{-7}\text{ m}[/latex]

[latex]\displaystyle\nu=\frac{c}{\lambda}=\frac{3.0\times10^8\text{ m/s}}{6.20\times10^{-7}\text{ m}}=4.8\times10^{14}\text{ Hz}[/latex]

Step 3: Think about your result.

The value for the frequency falls within the range for visible light.

Summary

All waves can be defined in terms of their frequency and intensity.
c = λν expresses the relationship between wavelength and frequency.

Practice

Read the material on the link below and answer the questions as they come up:

http://www.absorblearning.com/physics/demo/units/DJFPh064.html

Review

Define wavelength.
Define frequency.
What is the relationship between wavelength and frequency?

Glossary

amplitude: The height of the wave, the distance between the crest and the trough
crest: The peak of the wave
frequency: Is the number of waves that pass a certain point in a specified amount of time.
trough: The low point of the wave cycle.
wavelength: The distance between two successive peaks.